
If you're reading this, then I figure I've got another five to ten minutes of your time. On this page we discuss what the rest of this two-dimensional family looks like. Put m on the horizontal axis and k on the vertical axis. The following table shows what you have on your site. The powers of k+1, Lame's and Binets extend infinitely.
| powers of 2 | Fibonacci | Lame' | Lame' | Lame' |
| powers of 3 | Jacobsthal | Dragon curves | ||
| powers of 4 | Binet | mine | ||
| powers of 5 | Binet | |||
| powers of 6 | Binet |
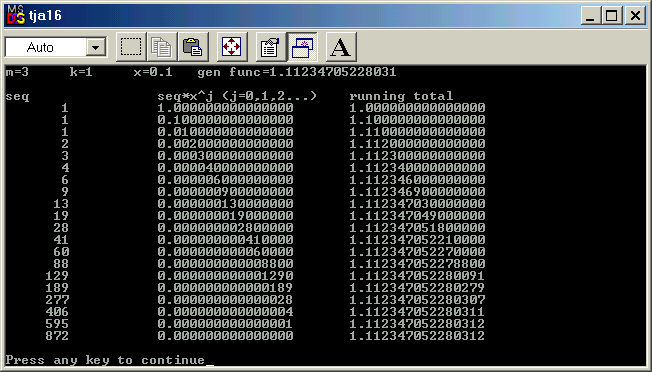
I tested my gen func for the Binet family:

None of the Binet's I saw included gen funcs, a glaring omission. A lot of them looked like somebody had spent less than an hour coughing them up and getting their name immortalized, without any reflection on what makes a sequence interesting. They're pretty much a mess and need some work.
I tested my gen func for the Lame' family:
The Lame's are cohesively presented and include gen funcs. Indeed, I made my notation consistent with it. I would think that we should add my sequence to these as a reference to indicate that there are a whole plane of these breeding rabbits.
As for Jacobsthal and dragon curve, the former is not identified as part of a family or a population problem, and the latter has no generating function. I think we should add a sequence for k=2, m=4 because 1,3,5,7,9,15 gets 9 matches, and we would want to show that there exists another possibility.

To reiterate, the best reason to include my sequence is that it is about as easy as generating functions get. A person can get his feet wet with these as opposed to those with more combinatorial content. Summer solstice woke me this morning, and I read Stanley for an hour. The clarity of his thought because he views enum comb through gen funcs is an epiphany.